- #1
DOTDO
- 7
- 0
Hi
I began to study the basics of QED.
Now I am studying Lagrangian and Hamiltonian densities of Dirac Equation.
I'll call them L density and H density for convenience :)Anyway, the derivation of the H density from L density using Legendre transformation confuses me :(
I thought because parameters of them are space-time components, it should be

But I found that this is related to the De Doner - Weyl Theory,
and the H density used in textbook is
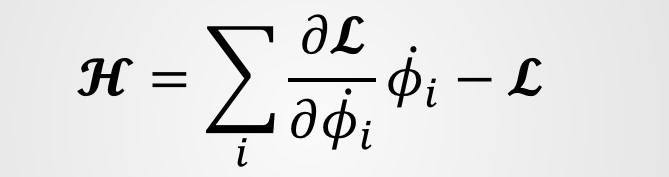
where the dot represents time derivative.So, my question is,
why we consider Legendre transformation on only time derivative of phi ?
Is it just 'defined' to consider energy of the system?
Then what does the covariant H density defined in the De Donder - Weyl theory mean?
I began to study the basics of QED.
Now I am studying Lagrangian and Hamiltonian densities of Dirac Equation.
I'll call them L density and H density for convenience :)Anyway, the derivation of the H density from L density using Legendre transformation confuses me :(
I thought because parameters of them are space-time components, it should be
But I found that this is related to the De Doner - Weyl Theory,
and the H density used in textbook is
where the dot represents time derivative.So, my question is,
why we consider Legendre transformation on only time derivative of phi ?
Is it just 'defined' to consider energy of the system?
Then what does the covariant H density defined in the De Donder - Weyl theory mean?