- #1
Aladdin123
- 16
- 0
Hi
So the problem I have is I want to get the equation of motion of a vibrating beam under a nonlinear "Point" force.
The equation would be like this for a distributed load (Which is not the case)
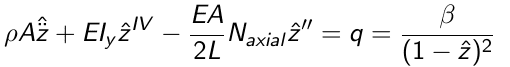
But I want the load to be at a point at x=L
So I have to options. Add an impulse dirac function multiplied by the beta (Causes numerical errors for my solver), or define the force as follows:
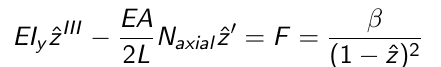
Thus

Which one is correct or if both wrong what do I do.
Also even if one of them is correct, any better options? (The above cases messes up my numerical solver)
Note: Obviously, z is a function of (x,t) and also beta is a function of (t)
So the problem I have is I want to get the equation of motion of a vibrating beam under a nonlinear "Point" force.
The equation would be like this for a distributed load (Which is not the case)
But I want the load to be at a point at x=L
So I have to options. Add an impulse dirac function multiplied by the beta (Causes numerical errors for my solver), or define the force as follows:
Thus
Which one is correct or if both wrong what do I do.
Also even if one of them is correct, any better options? (The above cases messes up my numerical solver)
Note: Obviously, z is a function of (x,t) and also beta is a function of (t)
Attachments
Last edited: