Baire Category Theorem .... Stromberg, Theorem 3.55 .... ....

[Math Amateur]

TL;DR Summary

The post concerns an aspect of Stromberg's proof of the Baire Category Theorem ... ...

I am reading Karl R. Stromberg's book: "An Introduction to Classical Real Analysis". ... ...

I am focused on Chapter 3: Limits and Continuity ... ...

I need help in order to fully understand the proof of Theorem 3.55 on page 110 ... ...Theorem 3.55 and its proof read as follows:

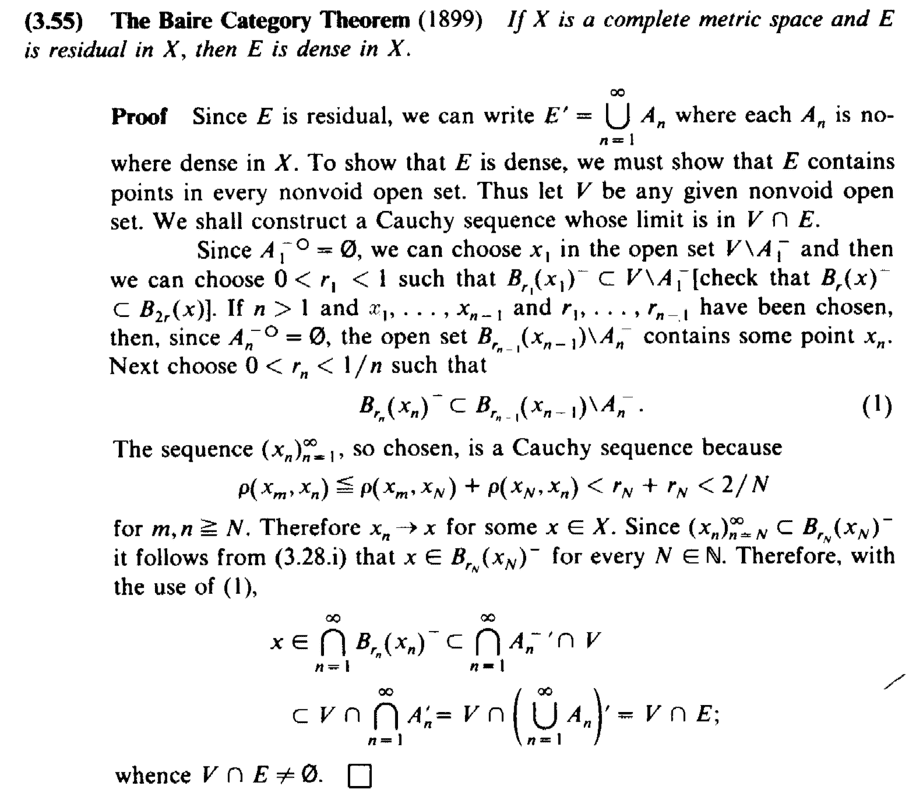At the start of the second paragraph of the above proof by Stromberg we read the following:

" ... ...Since ##A_1^{ - \ \circ } = \emptyset##, we can choose ##x_1## in the open set ##V## \ ##A_1^{ - }## and then we can choose ##0 \lt r_1 \lt 1## such that ##B_{ r_1 } ( x_1 )^{ - } \subset V## \ ##A_1^{ - }## [ check that ##B_r (x)^{ - } \subset B_{ 2r } (x) ## ] ... ...My questions are as follows:Question 1

Can someone explain and demonstrate exactly/rigorously why/how it is that ##A_1^{ - \ \circ } = \emptyset## means that we can choose ##x_1## in the open set ##V## \ ##A_1^{ - }## ... it seems very plausibly the case ... but ... how are we (rigorously) sure this is true ... ?
Question 2

How/why can we choose ##0 \lt r_1 \lt 1## such that ##B_{ r_1 } ( x_1 )^{ - } \subset V## \ ##A_1^{ - }## ... ?

... and why are we checking that ##B_r (x)^{ - } \subset B_{ 2r } (x)## ... ... ?

*** EDIT ***

My thoughts on Question 2 ...

Since ##V## \ ##A_1^{ - }## is open ... ##\exists \ r_1## such that ##B_{ r_1 } ( x_1 ) \subset V## \ ##A_1^{ - }## ...

... BUT ... how do we formally and rigorously show that ...

... we can choose an ##r_1## such that the closure of ##B_{ r_1 } ( x_1 )## is a subset of ##V## \ ##A_1^{ - }## ... ( ... intuitively we just choose ##r_1## somewhat smaller yet ... )

... and further why is Stromberg talking about ##r_1## between ##0## and ##1## ...?
Help will be much appreciated ...

Peter
==========================================================================================================================

The definitions of nowhere dense, first and second category and residual are relevant ... so I am providing Stromberg's definitions ... as follows:

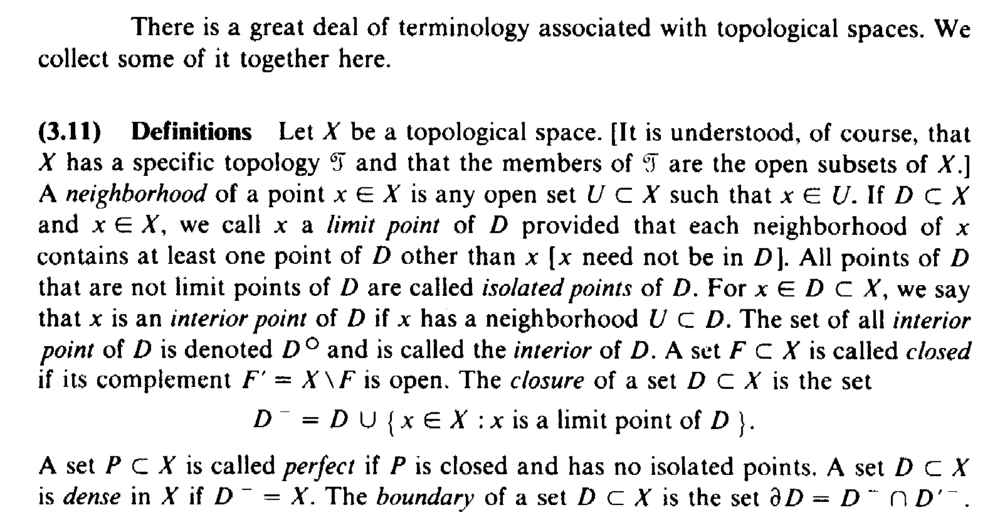
Stromberg's terminology and notation associated with the basic notions of topological spaces are relevant to the above post ... so I am providing the text of the same ... as follows:

Hope that helps ...

Peter

 

[andrewkirk]

On the first one:

Assume the opposite: that V\A1- is empty. Then A1- contains V, which is a nonempty open set and has interior points (since all points in an open set are interior). So A1- has interior points, but it is the closure of A1, and that contradicts the statement that A1 is nowhere dense. From this contradiction we conclude that V\A1- cannot be empty.

 

[andrewkirk]

On the second one, note that V\A1- is open because it is the intersection of open set V with the complement of A1-, which is open because A1- is closed. Since it is open, all its points are interior and in particular x1 is, so there exists some h such that the ball Bh(x1) is contained in V\A1- .

He requires r1<1 because a little further down he requires that rn < 1/n. That's how he makes the sequence Cauchy.

 

[Math Amateur]

Thanks so much for the help, Andrew

Just working through your posts now ...

Thanks again,

Peter

 

[Math Amateur]

On the second one, note that V\A1- is open because it is the intersection of open set V with the complement of A1-, which is open because A1- is closed. Since it is open, all its points are interior and in particular x1 is, so there exists some h such that the ball Bh(x1) is contained in V\A1- .

He requires r1<1 because a little further down he requires that rn < 1/n. That's how he makes the sequence Cauchy.

Thanks again for the help Andrew ...

... but ... just a clarification ...

... how exactly do we demonstrate rigorously .. given the ball ##B_h (x_1) \subset V## \ ##A_1^{ - }##

... we then have ##B_h (x_1)^{ - } \subset V## \ ##A_1^{ - }## ... ... ?Hoe you can help further ...

Peter

 

[andrewkirk]

Find an h' such that the open ball of radius h' is in that set. Then set h=h'/2. The closure of that ball will be inside the open ball of radius h' (as the closure is the set of points distant no further than h'/2 from the point, and that is a subset of the bigger open ball, set of points distance less than h' from the point), and hence will be in the required set.

This may relate to a tangential question you were asking earlier - something about checking the closures of balls with double or half the radius.