- #1
Math Amateur
Gold Member
MHB
- 3,998
- 48
I am reading Matej Bresar's book, "Introduction to Noncommutative Algebra" and am currently focussed on Chapter 1: Finite Dimensional Division Algebras ... ...
I need help with the proof of Lemma 1.25 ...
Lemma 1.25 reads as follows:
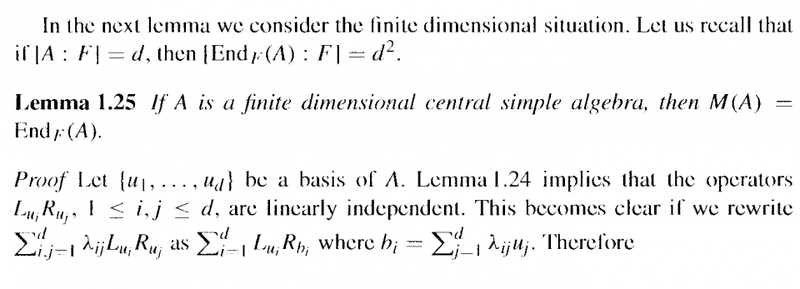

My questions on the proof of Lemma 1.25 are as follows:Question 1
In the above text from Bresar we read the following:
" ... ... Therefore ##[ M(A) \ : \ F ] \ge d^2 = [ \text{ End}_F (A) \ : \ F ]## ... ... "Can someone please explain exactly why Bresar is concluding that ##[ M(A) \ : \ F ] \ge d^2## ... ... ?Question 2
In the above text from Bresar we read the following:
" ... ... Therefore ##[ M(A) \ : \ F ] \ge d^2 = [ \text{ End}_F (A) \ : \ F ]##
and so ##M(A) = [ \text{ End}_F (A) \ : \ F ]##. ... ... "Can someone please explain exactly why ##[ M(A) \ : \ F ] \ge d^2 = [ \text{ End}_F (A) \ : \ F ]## ... ...
... implies that ... ##M(A) = [ \text{ End}_F (A) \ : \ F ]## ...
Hope someone can help ...
Peter
===========================================================*** NOTE ***
So that readers of the above post will be able to understand the context and notation of the post ... I am providing Bresar's first two pages on Multiplication Algebras ... ... as follows:

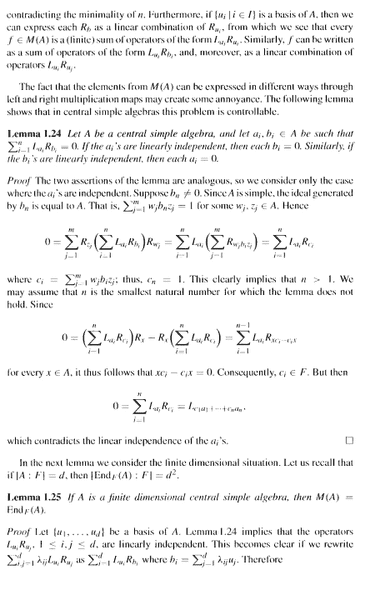
I need help with the proof of Lemma 1.25 ...
Lemma 1.25 reads as follows:
My questions on the proof of Lemma 1.25 are as follows:Question 1
In the above text from Bresar we read the following:
" ... ... Therefore ##[ M(A) \ : \ F ] \ge d^2 = [ \text{ End}_F (A) \ : \ F ]## ... ... "Can someone please explain exactly why Bresar is concluding that ##[ M(A) \ : \ F ] \ge d^2## ... ... ?Question 2
In the above text from Bresar we read the following:
" ... ... Therefore ##[ M(A) \ : \ F ] \ge d^2 = [ \text{ End}_F (A) \ : \ F ]##
and so ##M(A) = [ \text{ End}_F (A) \ : \ F ]##. ... ... "Can someone please explain exactly why ##[ M(A) \ : \ F ] \ge d^2 = [ \text{ End}_F (A) \ : \ F ]## ... ...
... implies that ... ##M(A) = [ \text{ End}_F (A) \ : \ F ]## ...
Hope someone can help ...
Peter
===========================================================*** NOTE ***
So that readers of the above post will be able to understand the context and notation of the post ... I am providing Bresar's first two pages on Multiplication Algebras ... ... as follows:
Attachments
-
 Bresar - 1 - Lemma 1.25 - PART 1 ... ....png27.6 KB · Views: 558
Bresar - 1 - Lemma 1.25 - PART 1 ... ....png27.6 KB · Views: 558 -
 Bresar - 2 - Lemma 1.25 - PART 2 ... ....png23.9 KB · Views: 673
Bresar - 2 - Lemma 1.25 - PART 2 ... ....png23.9 KB · Views: 673 -
 Bresar - 1 - Section 1.5 Multiplication Algebra - PART 1 ... ....png27.6 KB · Views: 521
Bresar - 1 - Section 1.5 Multiplication Algebra - PART 1 ... ....png27.6 KB · Views: 521 -
 Bresar - 2 - Section 1.5 Multiplication Algebra - PART 2 ... ....png29.5 KB · Views: 702
Bresar - 2 - Section 1.5 Multiplication Algebra - PART 2 ... ....png29.5 KB · Views: 702
Last edited: